Hello there! Today I?m showing you how to quickly and effectively simplify radicals of any index by hand. What?s the best part about this method? It?s (almost) foolproof?so long as you have a solid set of multiplication skills. Which, of course, I?m sure you do! Let?s get started!!

Capitalizing on the Fundamental Theorem of Arithmetic
There are very few theorems in mathematics that get to wield the word Fundamental in their name, and so, as you can imagine, they are some of the most useful theorems we have.
The Fundamental Theorem of Arithmetic falls nothing short of its powerful name. In short, the theorem says that every real number can be expressed as a product of its prime factors, and furthermore that product is unique to that number. That?s why the Fundamental Theorem of Arithmetic is also known as the Unique Prime Factorization Theorem.
It turns out that we can really capitalize on these unique prime factorizations to simplify our math. By replacing our number with its prime factorization not only do we take the guess-work out of simplifying radicals, we also greatly simplify our computations. Win-win!
How to find the Prime Factorization
Of course, in order to substitute our number for its prime factorization, we need to first find the prime factorization! This can easily be done by making a factor tree for your number.
To make a factor tree, simply begin by writing down the number you wish to factor and add two ?branches? below the number.
On the branches write down two whole number values that multiply to make your number (just don?t write down the factors 1 and your number, because that?s not going to be very helpful!).
For instance, if I want to simplify the square root of 60, I first need to know the prime factorization of 60. To do that I?ll write down 60, and below it I right two factors of 60, such as 6 and 10.
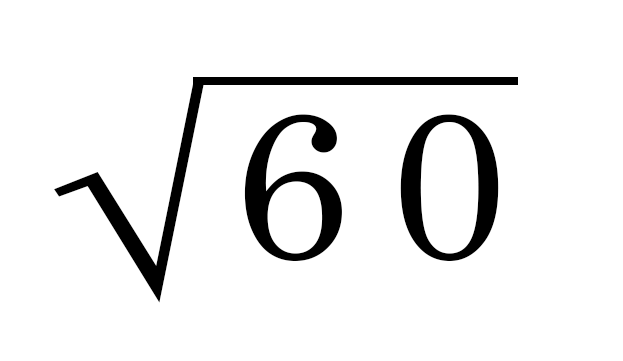
First replace 60 with the prime factorization we found above.
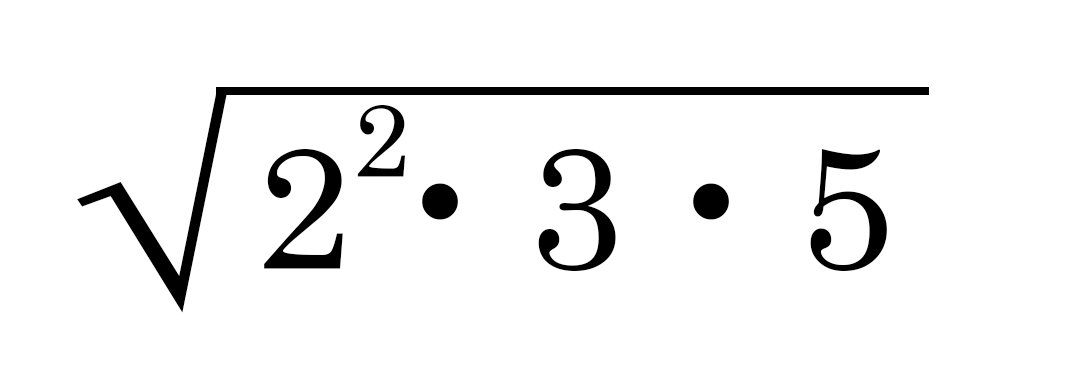
Next, split the radical into separate radicals for each factor.
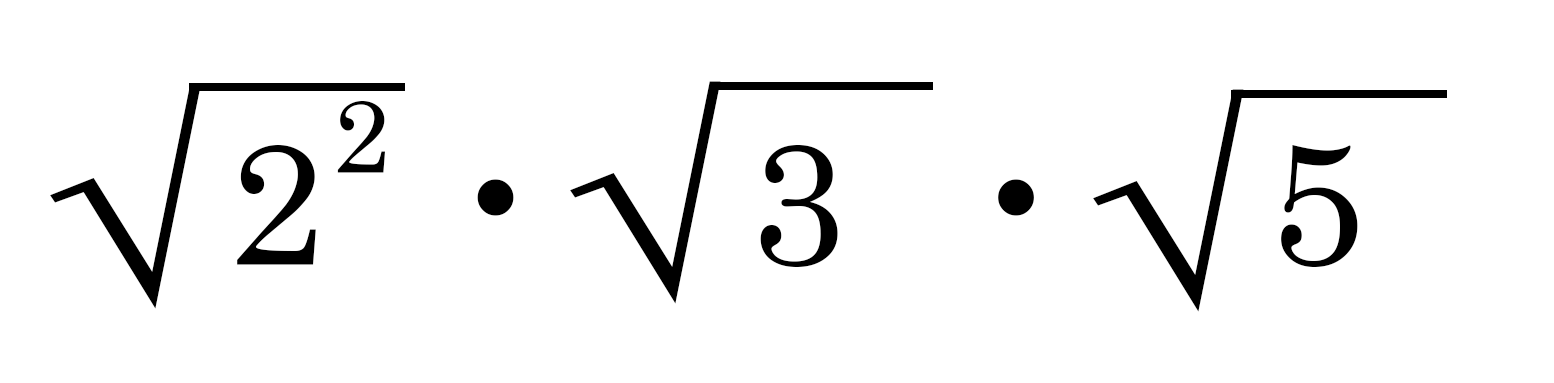
When working with square roots any number with a power of 2 or higher can be simplified. In this problem that means that the 2-squared can be simplified.
Since square roots and powers of 2 are inverses of each other, the square root of 2-squared simply equals 2.
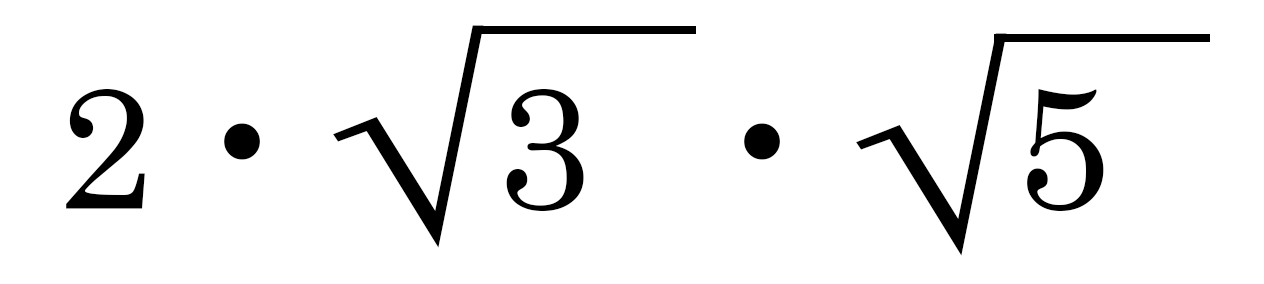
That?s all we can simplify in this problem, so let?s recombine our square root 3 and square root 5 back into one radical using multiplication.
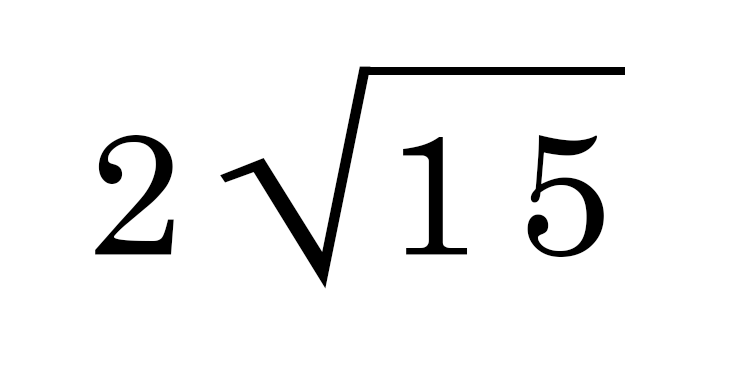
What about more difficult radicals?
That was a great example, but it?s likely you?ll run into more complicated radicals to simplify including cube roots, and fourth roots, etc. For a more in-depth explanation and some more examples, check out these tutorials from the Math Hacks channel!
How to Simplify Cube Roots by hand
Subscribe to Math Hacks
How to Simplify Radicals with Variables
Subscribe to Math Hacks on YouTube
Need More Math Help??
- Check out Math Hacks on YouTube for more hands-on math tutorials covering popular topics from Algebra through Calculus.
- You can find a number of interesting math topics and problems right here on Medium, just click that follow button!
Thanks for joining me!
? Brett
Instagram | Facebook | Twitter