During a Radio Five Live interview in 1998, UK school standards Minister Stephen Byers was asked: What is 7 times 8? He gave the answer 54. His erroneous answer prompted personal ridicule across the national media and helped push sentiment to make memorization of the 12 x 12 times table by age nine an English school standard.
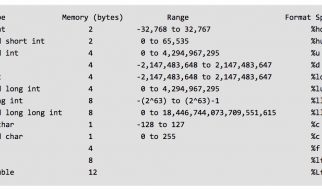
When you look at this table, does the ?54? seem out of place?
There are certainly valid psychological reasons for answering simple questions incorrectly under pressure. But let?s imagine Minister Byers had the opportunity and wherewithal to think for a moment before answering. As a hypothetical exercise, here are four possible answers he could have given. Which of them do you think might have inspired a sense of confidence in his abilities?
a) ?54?
b) ?This is a tricky one. But I remember being taught the mnemonic ?5 6 7 8.? So 7×8 is 56?.
c) ?This is a tricky one. But I do have in my head that 7×7 is 49, so 7×8 is seven more than this: 56.?
d) ?56″
It could be that many people would say response d) inspires the most confidence: ?Just know your math facts.? If your sole experience and expectation of mathematics is computation and computation facts, then speed facility is the key to success for that view of the mathematics. ?Let?s not deny our nine-year-old lads and lasses the opportunity to succeed.? Many folk further argue that practicing memorization promotes neural plasticity, among other things, and there does seem to be evidence that memorizing arithmetic facts for facility affects the hippocampal region of the brain, the region responsible for, well, memorizing facts.
So if computation is your primary view of mathematics and the memorization one conducts for success in it is conducted with joy and ease and fun-regular practice, then go for it. Just keep it joyful I say! And there are many pleasant ways to help young students develop their understanding of numbers and have arithmetic facts at ready hand. All is actually fine and good, despite the vehement debates about ?rote memorization? on the internet. Again, my only advice/wish: Always keep sight of joy.
Well, all is fine and good up to a point.
Those debates seem to be embedded in the context of early grade mathematics ? about memorizing multiplication tables, in particular ? with the stated goal of improving facility for upper-elementary arithmetic algorithms and the splattering of arithmetic that might come up in even later grades. We seem to forget that our young students are on a thirteen-year journey of mathematics, K through 12, that is. And the bulk of those years will not be focused on arithmetic computation. (Senior year mathematics is not about doing exceptionally long multiplication problems.)
Here?s my stance on the memorization debate, with regard to the upper-school mathematics of grades 8 through 12, and college math too:
I would be most impressed if Minister Byers answered c).
I say that fumbling is okay. Having the confidence to try recovering from a fumble, and to find success to boot, is admirable! Answer c) would say to me that the Minister, so many years since his schooling, has maintained an agility with number play and is thinking like a mathematician. Mathematics, in fact, could well be defined as the study of and play with structure and so c) demonstrates precisely the art and practice of doing mathematics. It is the mathematics I practice as a mathematician and the mathematics high-school and college students will experience.
***
There is too much content in the high school curriculum to memorize. (Well, some students do work to memorize it all, but I am not sure if that approach is joyful.) Consider for example, trigonometry, you know, the sine, cosine, and tangent of angles.
In a pre-calculus class one starts to play with trigonometric identities. And there a many of them! Here are twenty five your child will likely encounter ? and they are scary! (One equation is really three strung together.)
Where?s the call ?Our student need to know their trig identities by age 16.??
Of course, as a high school teacher, I don?t want students to memorize all these rules (and I, as a mathematician, don?t want them my head either: they are ugly and hard!)
So what do I want my students to do and what do I personally do as a mathematician?
We take inventory. That is, we take stock of what we already have in store and look to see what we can create from what we have. It is not an accident that the words inventory and invent are tied together.
The following diagram shows how students can reduce the task ?memorizing? this list to
a) recognizing five formulas as already in stock ? they are clear consequences of our picture of what trigonometry is,
b) recognizing that the bulk of formulas in the list follow as consequences of the three definitions and just two identities.
The five identities already ?in stock? and the three definitions and two identities needed to fill in the missing parts.
There are many trigonometric identities out there, many more than twenty-five. And after a class discussion like the one above I would be confident my high-school students could handle previously unseen identities. Just as I have hope that if Stephen Byers answered c) to the multiplication fact 7 x 8 = 56, he might have the agility and confidence of mind to see why 7 x 7 is mighty close to being 56 too. (How close?) Or should we have students memorize their fractional multiplication tables?
So would it be inappropriate to help prepare middle school students for this type of high school thinking?
Suppose, in a buoyant class discussion, we drew a 10 x 10 multiplication table on the board and asked students to
a) circle all the multiplication facts they think are easy and already have in their heads (the multiples of 1, the multiples of 10, 3 times 3, and so on)
b) observe that, since the table is symmetrical, there are only 55, not 100, multiplication facts to sort out. Phew!
c) discuss strategies for swiftly getting to the multiplication facts they find difficult and discuss what seems to make them difficult.
Perhaps in such a discussion the tricky products might naturally start to stick too.
***
All conversations require context. An exchange about the level of math-fact inventory necessary for good mathematical invention needs context too. Invention for what mathematics? What view of mathematics? What grade levels? Our students will experience a full range of mathematical structures in their K-12 journeys ? computation and arithmetic, geometry, algebra, modeling, and analysis and calculus ? each requiring a shift in mathematical maturity for handling the concepts involved. Can we keep that in mind?
But perhaps the memorization debates are not about mathematics per se, but the benefits of practicing memorization in and of itself, with the mathematics classroom just being one arena in school for doing so: recite all 144 multiplication facts in math class, all 50 state capitals in social studies class, passages from Macbeth in English class, and so on. Or perhaps it is a blend of both motivations: developing instant recall of multiplication facts has our youngsters practice memorization skills while building some mental inventory of K-5 computation facts at least. A win-win if you will.
I find it hard to decipher the motivation of many of the pieces I read on the internet about memorization in the mathematics classroom. I am usually unable to comment simply because I don?t know the underlying context of the argument.
But whatever the case presented for certain mathematical practices shall be, all will be good if we make sure the practice is true to mathematics, attends to the whole story of mathematics, and, most important, is conducted with joy for one and all. Push-ups might be necessary every now and then, for sure, and that is fine once we?ve played with mathematics for a while and know why we want to do push-ups. We just can?t let exercises be the sole defining mathematical experience for our students. (Many, like me, worry that a ?drill? approach to memorization falls into this trap.)
How and when we expect students to know or figure that 7 x 8 is 56 will always be up for debate, I am sure. Hopefully we can be clear and explicit in future conversations about which aspects of the K-12 mathematics journey we are speaking to. And as we reflect on the education system, let?s also be sure to acknowledge the work our educators already do. Right now they work hard to bring the meaning, relevance, and joy of mathematics to our students ? wonder, awe, practical use, push-ups and all. Their talents are remarkable, they strive for the best for our students, and all is for mighty good.
Three End-Notes
1. Just to complete my thoughts. Answer a) would perturb me too if the Minister stuck with that answer after some consideration. And answer b) would disappoint me. Sure, cute mnemonics are indeed cute, but they are superficial and usually don?t tend to attend to any issue of structure or meaning.
2. As a mathematician I balk at the idea of even getting the two remaining angle sum formulas in my head: they are just not beautiful! I thank Swiss mathematician Leonhard Euler who, two-and-a-half centuries ago, discovered a stunning formula that essentially reduces the entire study of trigonometric relations to the powers of a single special number e. I have only that one beautiful formula readily in my brain.
3. I linked to only a sparse number of internet pieces about the role of memorization in the mathematics classroom, far from representative of the full range of content in debate. Your own internet search on the matter will readily reveal so much more.
?L?/
