Thinking Like a Mathematician
Why politicians move to the center
In microeconomics and game theory, the median voter theorem states that
?In a majority rule voting system, the candidate/party most preferred by the median voter will be elected?
In other words, the favorite candidate of the person in the middle of the probability distribution picks the winner of the election. The prediction of the model, therefore, is what intuitively seems questionable (given today?s politics), namely that:
Candidates will position themselves around the center.
The theorem rests on two core assumptions:
- That candidates/parties may be placed along a one-dimensional political spectrum; and
- Voters? preferences are single-peaked, meaning voters have one alternative they prefer over the other;
A Simple Voting Model
The principle of the median voter theorem is successfully illustrated in a lecture by Yale economist Ben Polak which is available for free here, with notes here. His very simple model goes as follows:
A simple voting model (Polak, 2007)Two candidates choose their positions along a political spectrum from 1-10:_ _ _ _ _ _ _ _ _ _1 2 3 4 5 6 7 8 9 10Imagine perhaps positions 1-3 being left-wing positions and positions 8-10 being right-wing positions. Assume that there are 10% of voters in each position (uniform distribution). Assume that voters vote for the closest candidate to their position. If there is a tie, votes are split 50% / 50% between two candidates.
The question for both candidates is where they should position themselves along the political spectrum 1?10 in order to maximize their shares of the vote.
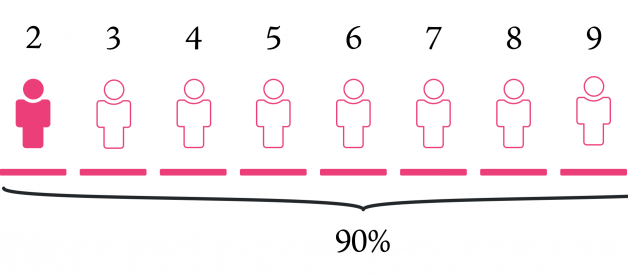
First, as candidate 1, let us assume candidate 2 places himself at the extreme left, in position 1. The question we need to ask ourselves is then whether position 2 is a better strategy for maximizing our share of the vote. Formally, we?re asking if u?(2,1) > u?(1,1). Considering each position potentially holds 10% of votes and if both choose the same position, we split the market, we know that if both choose position 1, (1,1), we both get 50% of the votes. If candidate 1 chooses 2 however, (2,1), candidate 2 gets the 10% of votes in position 1, but we get the remaining 90% of votes in positions 2?10 because as the result of our assumption that voters vote for the closest candidate:
u(1,2): Voter shares if candidate 1 chooses position 1 when candidate 2 chooses position 2
We can conclude that if candidate 1 chooses position 1, choosing 2 is better for us than choosing 1 is (90% vs 50% of votes).
Next, again as candidate 1, let us assume that candidate 2 places himself at the second most extreme left, in position 2. Is choosing position 2 still strictly better for us than choosing position 1? Formally, we?re asking whether u?(2,2) > u?(1,2). Immediately, since both are choosing the same position (2,2), we know that our payoff for choosing position 2 is 50% in this scenario. If we choose position 1 against position 2, we find us in the inverse of scenario in the figure above and can expect a payoff of 10%. We must therefore conclude that if candidate 2 chooses chooses position 2, we are still better off choosing position 2 (50% > 10% of votes).
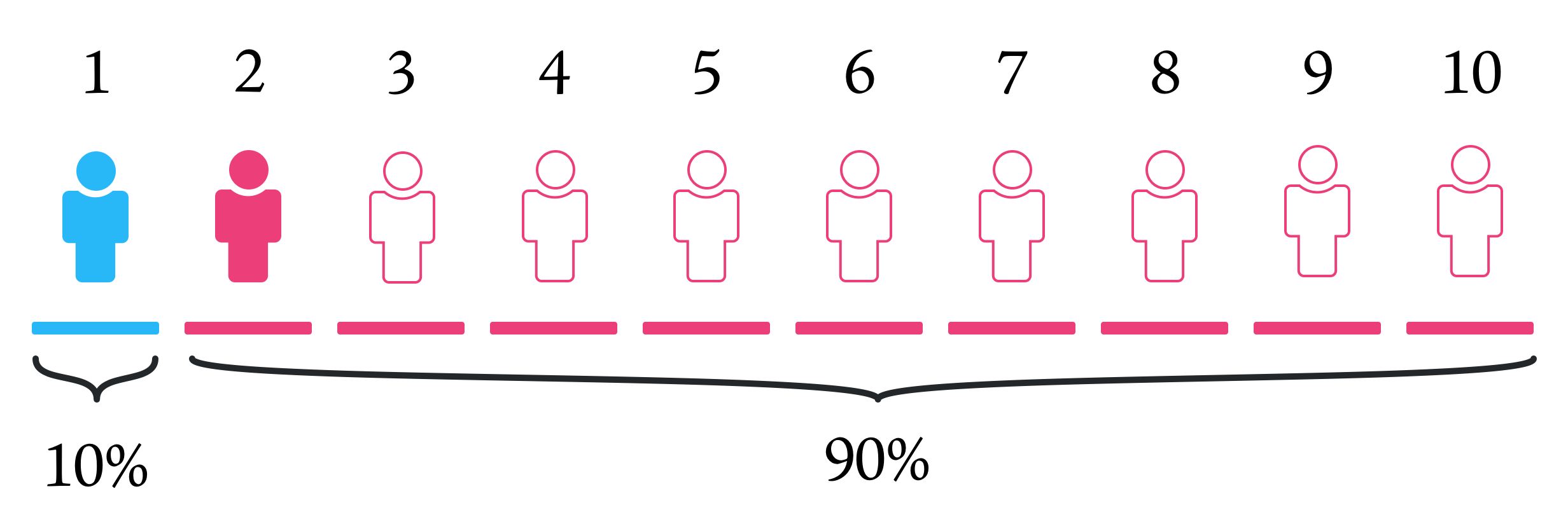
Next, again as candidate 1, let us assume that candidate 2 places himself at the third most extreme left, in position 3. Is choosing position 2 still strictly better for us than choosing position 1? Formally, now we?re asking whether u?(2,3) > u?(1,3). In the first scenario, we get all the voters in position 1 + all the voters in position 2 (20%), while candidate 2 gets all the voters in positions 3?10 (80%). In the second scenario, we get all the voters in position 1 + half the voters in position 2 (since we are equally far from position 2 as candidate 2 is, 15%). Either way, we see that position 2 is still strictly better for us than position 1 (20% > 15% of votes):
u(1,3): Voter shares if candidate 1 chooses position 1 when candidate 2 chooses position 3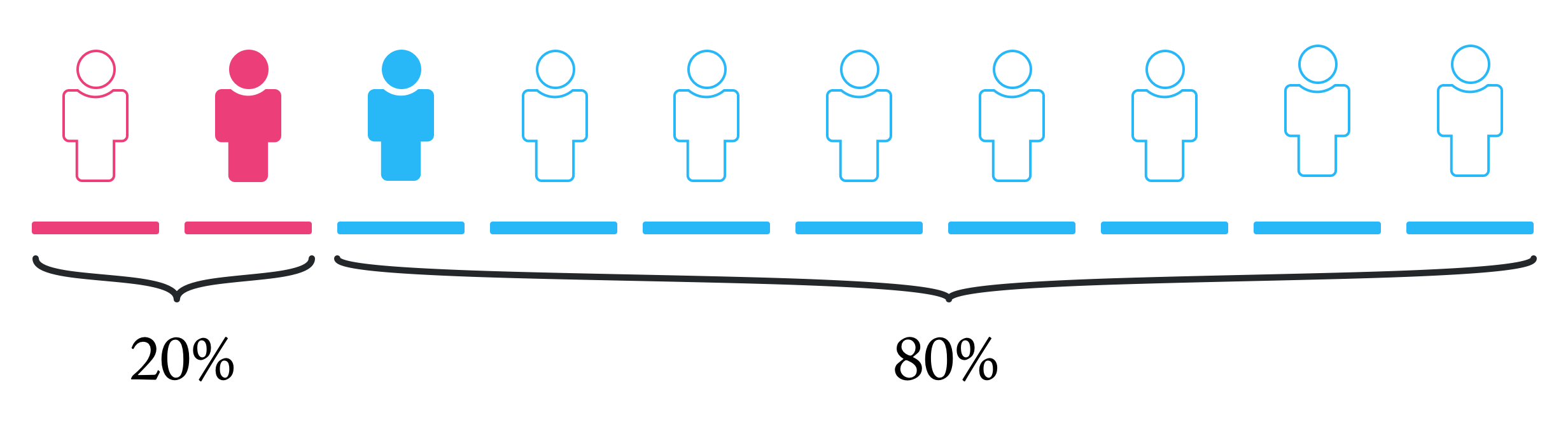
u(2,3): Voter shares if candidate 1 chooses position 2 when candidate 2 chooses position 3
Continuing for all strategies against us choosing position 1, we will find that position 2 always yields a higher payoff, i.e. position 2 strictly dominates position 1. We can hence eliminate position 1 as a viable strategy, since it is never better than position 2. By considerations of symmetry, we can do the same for position 10 vs position 9:
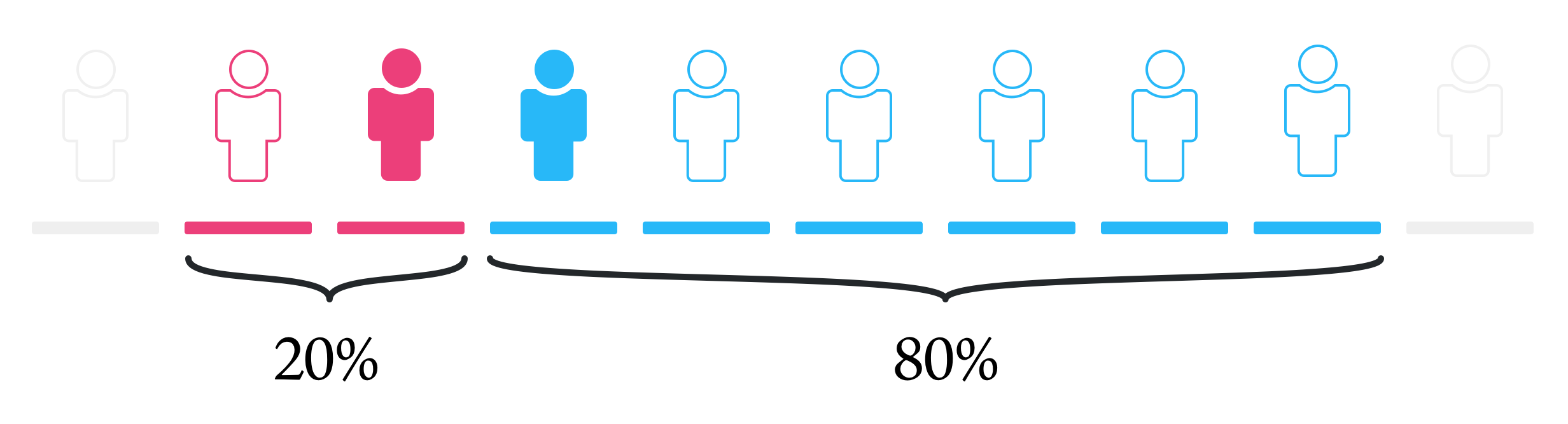u(2,3) in the reduced political spectrum
Having eliminated positions 1 and 10 as viable strategies for maximizing our number of votes, we can next perform a similar analysis, but now with a reduced model consisting of positions 2?9, since 1 and 10 have been eliminated. Unexpectedly, an analysis of position 2 vs 3 (9 vs 8) will yield the same result, and so on. In the end, following the same logic, our model will have reduced to two viable positions, 5 and 6, call them ?left and right?.
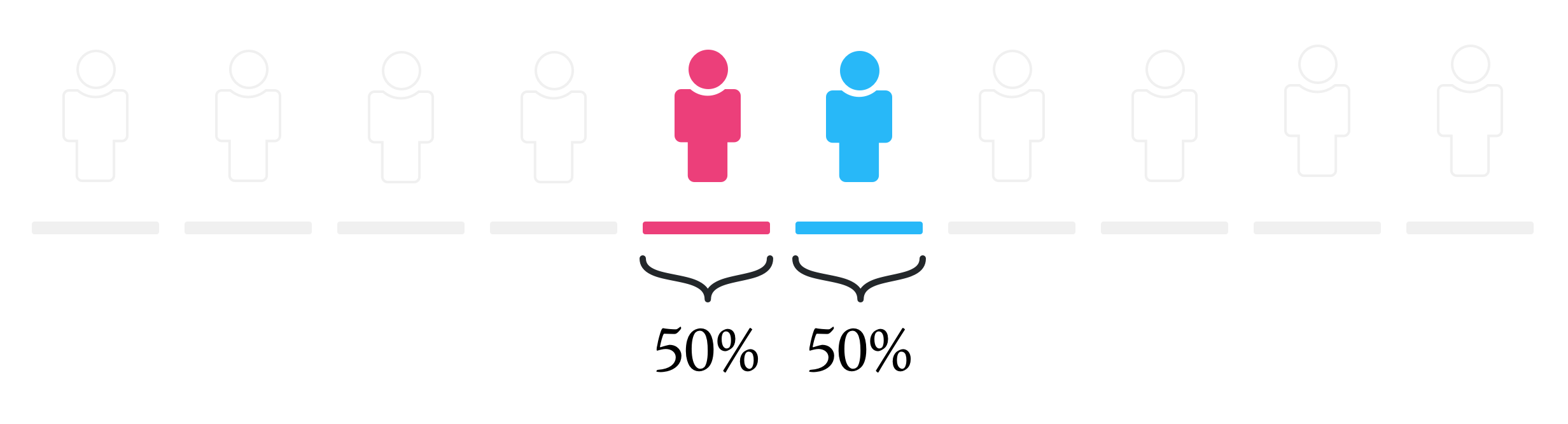u(5,6) = u(6,5) in reduced political spectrum after eliminating dominated strategies
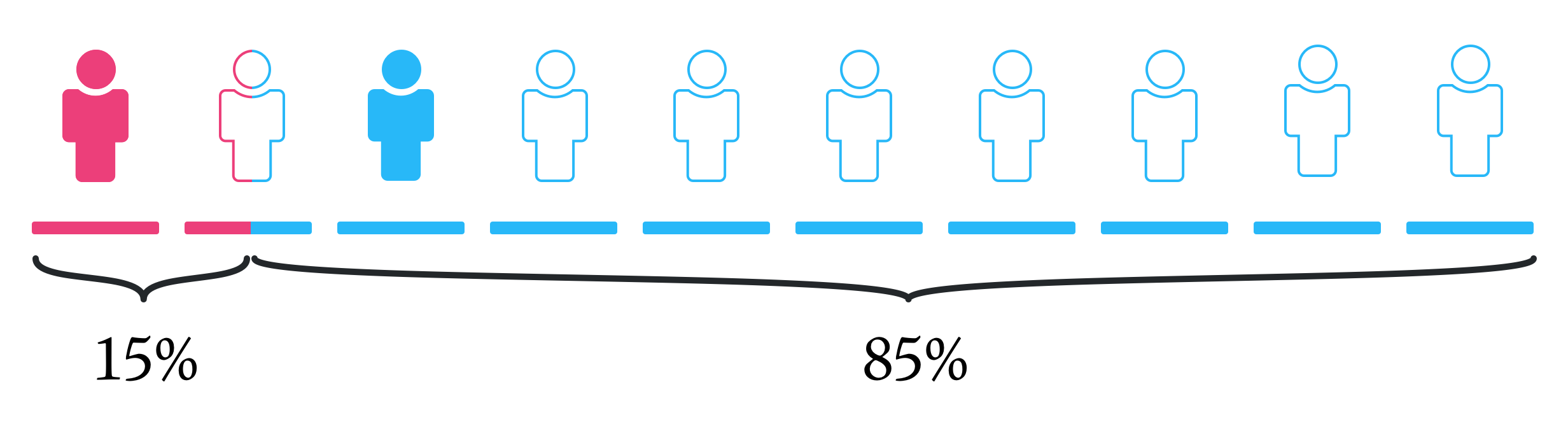
An analysis of this reduced model will find that if we expect candidate 2 to position himself on the right, it is rational for us to position ourselves on the left. If we expect candidate 2 to position himself on the left, it is rational for us to position ourselves on the right:
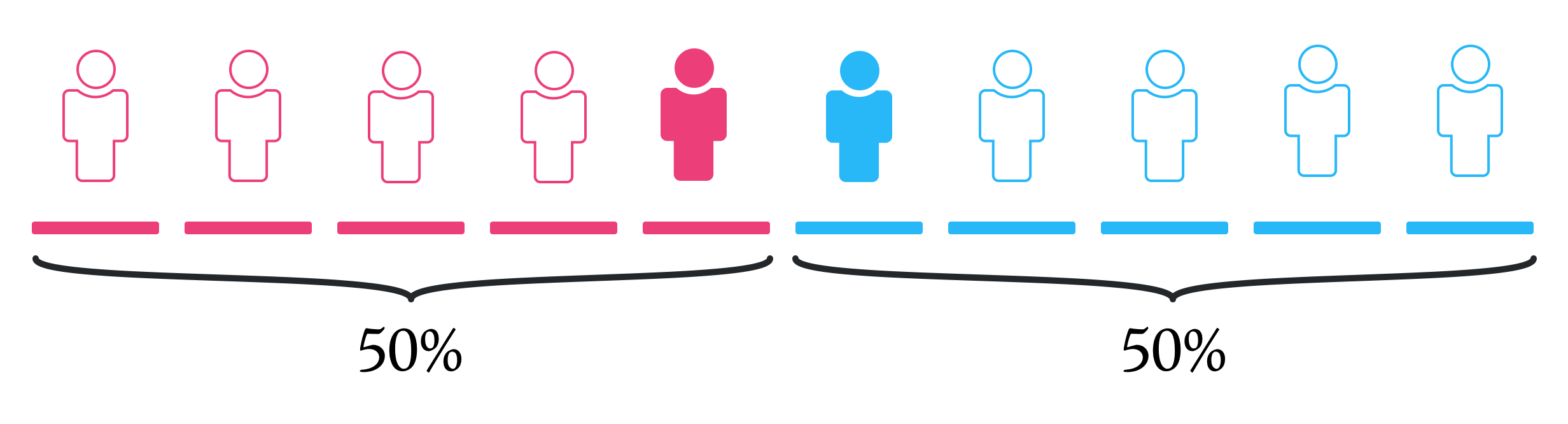u(5,6) = u (6,5): Voter shares if candidate 1 chooses position 5 and candidate 2 chooses position 6
Our finding, in other words is the prediction of the median voter theorem, namely that:
Candidates will position themselves around the center.
Clean as it looks, as with any model, our model depicting the essence of the median voter theorem is ripe with limitations, including that:
- Voters are in reality not evenly distributed (10% at each position);
- There are often more than two candidates in an election;
- Real candidates cannot simply ?position? themselves, their positioning has to be believable;
- Even in referendums, there is often more than one issue being voted on;
and so on.
Left: Harold Hotelling (1895?1973). Right: Hotelling?s 1929 paper ?Stability in Competition? in which the original form of the famous median voter theorem was first derived.
History
The dynamics and predictions of the median voter theorem made their first appearance in economist Harold Hotelling?s 1929 paper Stability in Competition, in which Hotelling in passing notes that political candidates? platforms seem to converge during majoritarian elections. His paper regards the positioning of stores by two sellers along a line segment, in which buyers are uniformly distributed. The prediction of his model, now known simply as ?Hotelling?s Law? is that in many markets it is rational for producers to make their products as similar as possible, the so-called ?principle of minimum differentiation?.
The formal analysis of the principle of Hotelling?s Law in majority voting systems was provided in a related 1948 paper entitled On the Rationale of Group Decision-making by economist Duncan Black. Anthony Downs, inspired by Adams Smith, further expanded on Black?s work in his 1957 book An Economic Theory of Political Action in Democracy.
This essay is part of a series of stories on math-related topics, published in Cantor?s Paradise, a weekly Medium publication. Thank you for reading!
