If you calculate both values, you?ll see it?s $2 in both cases. But 25% of $8 is much easier to do in your head, which is why it?s useful to keep in mind that percentages are ?reversible? like that.
This article is motivated by an innocent Reddit question that spilled over to Twitter:
If you dare to read the thread attached to that tweet, you might lose some faith in humanity. Do it at your own risk.
Back to the matter at hand: 8% of 25 = 25% of 8. Why?
If you think about it for a second it might be obvious. But I have to admit, that I actually had to. Think about it, that is.
So here?s why this works.
One of the reasons why we?re not quick to see the above equality is that the % sign assigns a meaning in our minds, much like a unit does. If something is a number with miles/h attached, we know it?s a speed and understand its meaning in an equation.
Similarly, taking a percentage of some quantity is taking a fraction of that quantity. Our mind assigns a meaning to the percentage number, and the whole quantity. A swap in semantics does not make sense, so we don?t suspect that it is possible in the math.

But at the level of abstraction ? working with just numbers as opposed to cakes, pizzas, dollars, or whatever we?re taking fractions of ? we can see it.
To take a percentage is to multiply
If we want to take a percentage of something, we multiply.
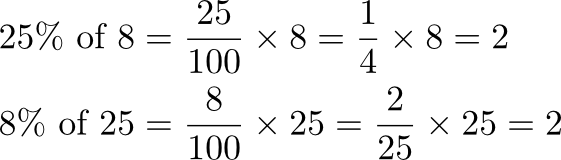 Calculating percentages
Calculating percentages
Remembering that 25/100 is just 25 1/100 we can also decide to write down all three factors:
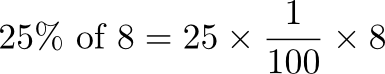 Expressing a percentage as three factors
Expressing a percentage as three factors
Now that is a multiplication of three numbers, and we know that the order in which we multiply does not matter.
We can re-order the numbers in different ways, and each ordering corresponds to a different re-phrasing of the same quantity in percentage notation. The result stays the same.
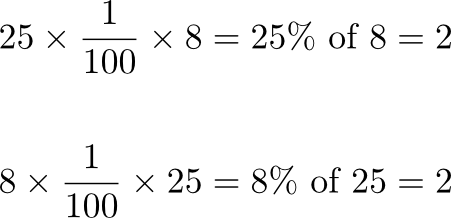 We?re just re-ordering
We?re just re-ordering
We can also find an ordering of factors that reminds us that % just means ?by hundred? and therefore a %-value technically has a magnitude of its own, and need not be related to some ?whole?.
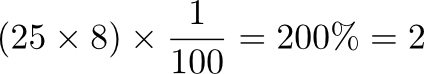 Percentages have a magnitude of their own
Percentages have a magnitude of their own
Admittedly %-notation is not intended for that purpose, but if you ever need to calculate 15% of 20 in your head, (15×20)% = 300% = 3 might very well be the fastest route.
So there. Next time you calculate percentages in your head, there?s one more little trick you can apply.
PS: Don?t read the tweet?s comments. You might read the comment of someone you know, and it will be embarrassing. Just don?t.